The physics of a singing saw

The sound of the singing saw has been a part of folk music traditions around the world since the early 19th century, due to cheap, flexible steel. The instrument was made from bending a metal hand saw and bowed like a cello and was popular in the early 20th century, but has seen a resurgence thanks to social media.

The unique mathematical physics of the singing saw may hold the key to designing high quality resonators.

A team of researchers from the Harvard John A. Paulson School of Engineering and Applied Sciences used a singing saw to demonstrate how the geometry of a curved sheet could be changed to create high-quality, long metal.

L Mahadevan, the Lola England de Valpine Professor of Applied Mathematics, said that their research offers a robust principle to design high-quality resonators independent of scale and material.

The research is published in a journal.

The singing saw is the only musical instrument that works like a singing saw.

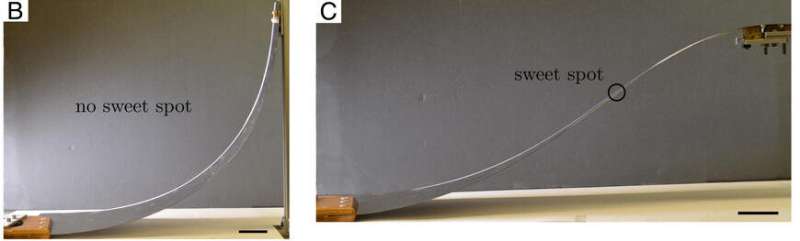
Petur Bryde, a graduate student at SEAS and co-first author of the paper, said how the singing saw sings is based on a surprising effect. A dull sound is created when the energy is lost through the boundary where it is held. If you curve it into a J-shape, the result is the same. If you bend the sheet into an S-shape, you can make it vibrate in a small area, which produces a long- lasting tone.

The sweet spot is created by the geometry of the curved saw, and physicists call it a confined area on the sheet which vibrates without losing energy at the edges.

The geometry of the S-curve doesn't matter. It could be an S with a big curve at the top and a small curve at the bottom.

The underlying mechanisms have remained a mystery, despite the fact that musicians and researchers have known about this robust effect of geometry for some time.

An analogy to very different class of physical systems was found by the three men. Most often associated with quantum physics, topological insulators are materials that conduct electricity in their surface or edge but not in the middle and no matter how you cut these materials, they will always conduct on their edges.

In this work, we drew a mathematical analogy between the acoustics of bent sheets and quantum and electronic systems.

The researchers found that the sweet spot of singing saws was governed by a topological parameter that could be computed and which relied on two opposite curves in the material. The sweet spot behaves like an edge in the saw.

By using experiments, theoretical and numerical analysis, we showed that the S-curvature in a thin shell can localize topologically-protected modes at the sweet spot.

The researchers found that they could tune the mode by changing the shape of the S-curve, which is important in applications such as sensor.

The researchers want to explore modes in doubly curved structures.

More information: Suraj Shankar et al, Geometric control of topological dynamics in a singing saw, Proceedings of the National Academy of Sciences (2022). DOI: 10.1073/pnas.2117241119 Journal information: Proceedings of the National Academy of Sciences Citation: The physics of a singing saw (2022, April 22) retrieved 22 April 2022 from https://phys.org/news/2022-04-physics.html This document is subject to copyright. Apart from any fair dealing for the purpose of private study or research, no part may be reproduced without the written permission. The content is provided for information purposes only.