Vacuum fluctuations break topological protection

A hallmark of so-called topological quantum states is that they are protected. In the case of the Hall effect, vacuum fluctuations can cause a breakdown of protection.

The German physicist Klaus von Klitzing spoke about the effect of the Quantized Hall Effect, which is dependent on fundamental constants and is not affected by the effects of the Semiconductor like impurities or interface effects. The Hall effect in two-dimensional electron gasses was quantised by him in 1980. The discovery of von Klitzing was made thanks to the unexpected robustness of the quantum Hall effect. He was working with materials that were notoriously plagued by flaws, yet he observed a clean quantization of the Hall Conductivity. The framework of the topological properties of electronic many-body states explains the fact that quantum systems can be protected against local perturbations. The Institute of Quantum Electronics now reports that protection can break down in unexpected ways. Experiments in which they established that exposing a quantum Hall system to the strongly enhanced quantum vacuum fluctuations of a tight cavity provides a novel and potentially general route to substantially modify quantum states can be found in Science. It might lead to new experimental capability, but could also cause unwanted interference in experiments combining two-dimensional materials and resonators.

Control by vacuum field engineering.

One of the most captivating aspects of quantum field theories is the empty space filled with vacuum fluctuations. The Lamb shift and the Casimir effect are examples of manifestations of vacuum fields. The work of the Faist group in which they determined the characteristics of vacuum-field fluctuations was the most recent example of direct detection of these fields. There were intriguing questions about whether vacuum fields could be harnessed to modify the properties of materials. In earlier work, a collaboration of ETH physicists found signs of magneto-transport when measuring in a Semiconductor material coupled to a Cavity. In these experiments the signatures of the modification depended on the sample itself and different physical samples had to be measured to compare situations in which the properties were modified by vacuum fields.

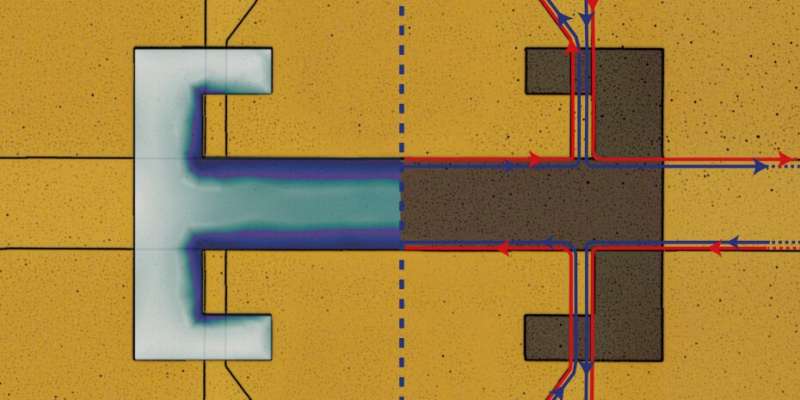
The Faist group has turned to a system in the integer quantum Hall regime, where topological protection ensures robustness and therefore removes some dependence on the specific sample used. Hall-bar samples were placed into the confines of an optical nano-cavity, with dimensions well below the wavelength of the light involved. A highly sensitive optical probe for quantum many-body states can be provided by such cavities. Over the past decade, the team around Faist and Giacomo Scalari, now an adjunct professor in the group, have been able to perfect split-ring cavities to reach record-high light. The tight sub-wavelength confinement enhances vacuum-field fluctuations, enabling both tweaking out their effect on matter and providing a route to vacuum-field engineering. There was a missing baseline in the previous work.

Out of step.

Appugliese and his team created and tested a reference sample without a cavity and a sample with a cavity on the same chip. They measured the staircase sequence in resistance first seen by von Klitzing. They saw deviations from the quantization in the sample with the cavity. They can be explained in a framework by their theory partner. In other words, the cavity opens up a channel through which an electron can be scattered, breaking the topological protection. Short-range interaction can't produce scattering.

Appugliese and colleagues put a metallic surface on the field inside the resonator, which was different from the sample. They show how vacuum-field-induced modification of the quantum Hall state can be done without reference samples. These results remind us that platforms combining two-dimensional materials and resonator structures could be prone to unwanted interference effects. This warning could also apply to situations without dental work. Thanks to the exact quantization they offer, Quantum Hall samples serve as a standard for electrical resistance and as a high-precision probe of fundamental constants. As free space is filled with vacuum fluctuations, it is possible that these fields limit the precision of such benchmarks.

More information: Felice Appugliese et al, Breakdown of topological protection by cavity vacuum fields in the integer quantum Hall effect, Science (2022). DOI: 10.1126/science.abl5818

Ileana-Cristina Benea-Chelmus and her co-workers measured the electric field correlation on the vacuum state. There is a DOI of 10.1038/s41586-019-1083-9.

The study was published in Nature Physics. There is a DOI titled: 10.1038/s41567-018-0346-y.

Journal information: Nature , Science , Nature Physics Citation: Vacuum fluctuations break topological protection (2022, March 4) retrieved 4 March 2022 from https://phys.org/news/2022-03-vacuum-fluctuations-topological.html This document is subject to copyright. Apart from any fair dealing for the purpose of private study or research, no part may be reproduced without the written permission. The content is provided for information purposes only.