The Chemnitz University of Technology has a PhD in technology.
There is a strategy for observing and manipulating the optical Berry phase in Mbius ring microcavities. They talk about how an optical Berry phase can be created and measured in Mbius rings. The first experimental proof of the existence of a variable Berry phase was presented.
The Mbius strip is interesting. A Mbius strip can be created by twisting the two ends of a strip of paper together. You realize that the ribbon only has one surface that can't be distinguished between inside and outside. In paintings by M.C. Escher, the Mbius strip has been used as a wedding ring or as a drive belt.
The optical ring is made of glass.
A closed band or ring is an important part of optoelectronics. They have not included Mbius strips and they are not made of paper, but are made of optical material. The rings are not centimeters in size. Light propagating in a micro ring causes optical resonances to occur The principle can be seen in a guitar string, which has different tones at different lengths and wavelength.
When the ring is a multiple of the wavelength of the light, there is an optical resonance. The ring is referred to as an optical ring resonator in these cases. When the ring's diameter is an odd multiple of half the wavelength of the light, it causes destructive interference. An optical ring resonator enhances light of certain wavelength and reduces light of other wavelength that isn't in the ring. The ring resonator is an optical filter that can be used to sort and process light. Today's data communication networks use optical ring resonators to process signals.
The Mbius strip has a light source.
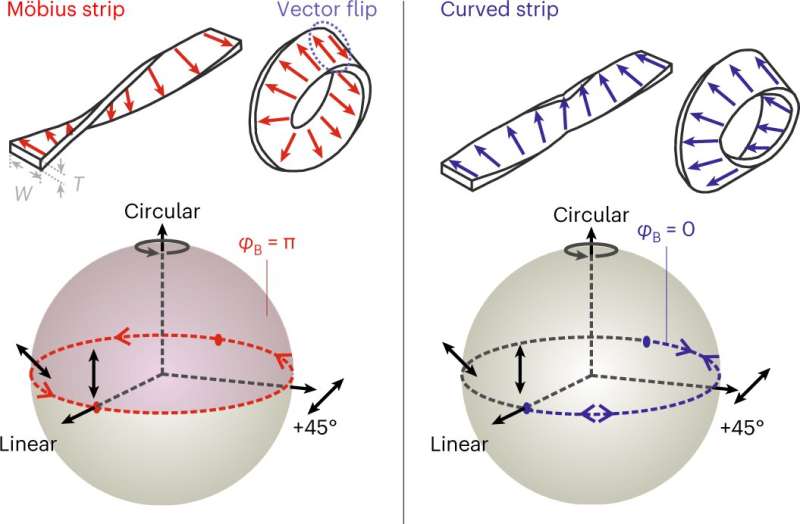
The property of light is important. Light can be either linear or circular. If light travels in an optical ring, it will stay the same at all points in the ring.
If a Mbius strip or better is used, the situation will change fundamentally. The geometry of the Mbius ring is important in understanding this case. The cross-section of a Mbius ring is usually a thin strip of paper with two edges longer than the other.
The Mbius ring has linearly polarized light. The Mbius ring has a long cross-sectional side that the polarization likes to align itself in. There is a huge difference between a normal ring resonator and this one.
Also, that's not the only thing. The optical resonances don't occur at full wavelength multiples that fit into the ring, but at odd multiples of half the wavelength, because of the twisting of the polarization. A part of the research group had predicted this effect in the past. This prediction is based on work by physicist Michael Berry, who introduced the Berry phase in 1983, describing the change in the phase of light.
It was the first evidence of its kind.
The Berry phase of light circulating in a Mbius ring is shown for the first time in the current article. Two rings with the same size were made. There are two rings, the first is a normal ring and the other is a Mbius ring. The Mbius ring has different optical resonances at different wavelength.
The results of the experiment go far beyond what had been predicted. The linear polarization is becoming increasingly elliptical. The resonances are not always at odd multiples of half the wavelength. The Mbius rings were made to find the reason for the deviation. As the Mbius strip became smaller, the degree of ellipticity in the ring became weaker.
When the width of the band decreases to that of its thickness, the special topological properties of the Mbius ring come into play. Changing the design of the band will allow for easy control of the Berry phase in Mbius rings.
New technological applications are opening up due to the new fundamental properties of optical Mbius rings. The Berry phase in Mbius rings can be used for all-optical data processing of classical bits as well as qubits.
The study of Berry phases in optical Mbius-strip microcavities was published in Nature Photonics.
Journal information: Nature Photonics
It was provided by the University of Technology.